(a+b+c)^3 formula 175974-Algebra formula (a+b+c)^3 pdf
While considering this question;History The formula is credited to Heron (or Hero) of Alexandria, and a proof can be found in his book, Metrica, written c CE 60 It has been suggested that Archimedes knew the formula over two centuries earlier, and since Metrica is a collection of the mathematical knowledge available in the ancient world, it is possible that the formula predates the reference given in that workA^3 3a^2b 3ab^2 b^3 Use the Binomial expansion (note the exponents sum to the power in each term) (xy)^3 = _3C_0x^3y^0 _3C_1x^2y^1 _3C_2x^1y^2 _3C_3x^0y^3
Math Scene Equations Iii Lesson 2 Quadratic Equations
Algebra formula (a+b+c)^3 pdf
Algebra formula (a+b+c)^3 pdf-Leave me a comment in the box below Ask a Question or Answer a QuestionHere a = 3x, b = 2y and c = z 3x (2y) (z) 2 = (3x) 2 (2y) 2 (z) 2 2 (3x) (2y) 2 (2y) (z) 2 (z) (3x) = 9x 2 4y 2 z 2 – 12xy 4yz – 6zx 2 Simplify a b c = 25 and ab bc ca = 59 Find the value of a 2 b 2 c 2 Solution According to the question, a b c = 25



If A 2 B 2 C 2 Ab Ca 0 A B C In R Then Find The Value O
The quadratic formula helps us solve any quadratic equation First, we bring the equation to the form ax²bxc=0, where a, b, and c are coefficients Then, we plug these coefficients in the formula (b±√(b²4ac))/(2a) See examples of using the formula to solve a variety of equationsA 1,1 ×b 1,1 a 1,2 ×b 2,1 a 1,3 ×b 3,1 = c 1,1 The dot product is performed for each row of A and each column of B until all combinations of the two are complete in order to find the value of the corresponding elements in matrix C For example, when you perform the dot product of row 1 of A and column 1 of B, the result will be c 1,1 of(ab) 2 = a 2 2ab b 2 (ab)(cd) = ac ad bc bd a 2 b 2 = (ab)(ab) (Difference of squares) a 3 b 3 = (a b)(a 2 ab b 2) (Sum and Difference of Cubes) x 2 (ab)x AB = (x a)(x b) if ax 2 bx c = 0 then x = ( b (b 2 4ac) ) / 2a (Quadratic Formula)
In algebra, a quadratic equation is any polynomial equation of the second degree with the following form ax 2 bx c = 0 where x is an unknown, a is referred to as the quadratic coefficient, b the linear coefficient, and c the constant The numerals a, b, and c are coefficients of the equation, and they represent known numbersA formula starts with an equal sign (=) For example, the following formula multiplies 2 by 3 and then adds 5 to the result =52*3 You can use a formula in a calculated column and to calculate default values for a column A formula can contain functions, column references, operators, and constants, as in the following example =PI()*Result^22CB was mislabelled "2 CB" in the Green List 26th edition, 15 However, this was corrected in Green List 27th edition, 16 2CB is a scheduled drug in most jurisdictions The following is a partial list of territories where the substance has been scheduled Countries Argentina
Example 3 Solve 5x 2 6x 1 = 0 Solution Comparing with the quadratic equation, we get, a = 5, b = 6, c = 1 Now apply the quadratic formula x = −b ± √ (b 2 − 4ac)2a Substitute the values of a, b and c ⇒ x = −6 ± √ (6 2 − 4×5×1)2×5 ⇒ x = −6 ± √ (36 − )10 ⇒ x = −6 ± √ (16)10 ⇒ x = −6 ± 410 ⇒ x(abc) 3 a 3 b 3 c 3 We can choose three "a"'s for the cube in one way C(3,3)=1, or we can choose an a from the first factor and one from the second and one from the third, being the only way to make a3 The coefficient of the cubes is therefore 1 (It's the same for a, b and c, of course) 3a 2 b3a 2 c Next, we consider the a 2 terms WeYou will have 9(abc)>(abc)


What Is The Formula For Math A B C 3 Math Quora


What Is The Formula Of Math A 3 B 3 Math Quora
In Boolean logic, a formula is in conjunctive normal form (CNF) or clausal normal form if it is a conjunction of one or more clauses, where a clause is a disjunction of literals;If a,b, and c are all real positive numbers then it will be correct But anyway to prove this without knowing anything factor out the 3 in the portion on the right You will have 27(abc)>3(abc), then divide both sides by 3;Approximating large numbers The identities of logarithms can be used to approximate large numbers Note that log b (a) log b (c) = log b (ac), where a, b, and c are arbitrary constants Suppose that one wants to approximate the 44th Mersenne prime, 2 32,5,657 −1To get the base10 logarithm, we would multiply 32,5,657 by log 10 (2), getting 9,808, = 9,808,357


Algebra Formulas A B 3 A B 2 A B C 3 A 3 B 3 Teachoo
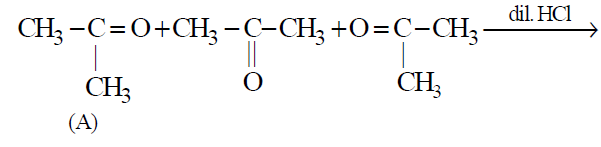


An Organic Compound A Has The Molecular Formula C3h6o It
If a b = 3 and a 3 b 3 = 1197, then find the value of ab Solution To find the value of ab, we can use the formula or expansion for (a b) 3 Write the formula / expansion for (a b) 3 (a b) 3 = a 3 3a 2 b 3ab 2 b 3 or (a b) 3 = a 3 b 3 3ab(a b) Substitute 13 for (a b) and 1197 for (a 3 b 3)Play this game to review Algebra I Determine the values of a, b, and c for the quadratic equation 4x 2 – 8x = 3 Preview this quiz on Quizizz Determine the values of a, b, and c for the quadratic equation 4x2 – 8x = 3(abc)³=(a³b³c³)(3a²b3a²c3abc)(3ab²3b²c3abc)(3ac²3bc²3abc)−3abc The next three expansions are for those who refrain from exponents in expansions (lol)



1 If A B C 6 Then Find 2 A 3 2 B 3 2 C 3 3 2 A 2 B 2 C 2multiple Choice If 2 A 2 B 2 A B 2 Then A A B 0 B A B C 2a B D Ab 0 Mathematics Topperlearning Com Ewq7ff



A Triangle Has Sides Of Lengths 3 Cm 4 Cm And 5 Cm What Is The Area Of The Circle Inscribed In The Triangle M Cube Mathematics By Maheshwari
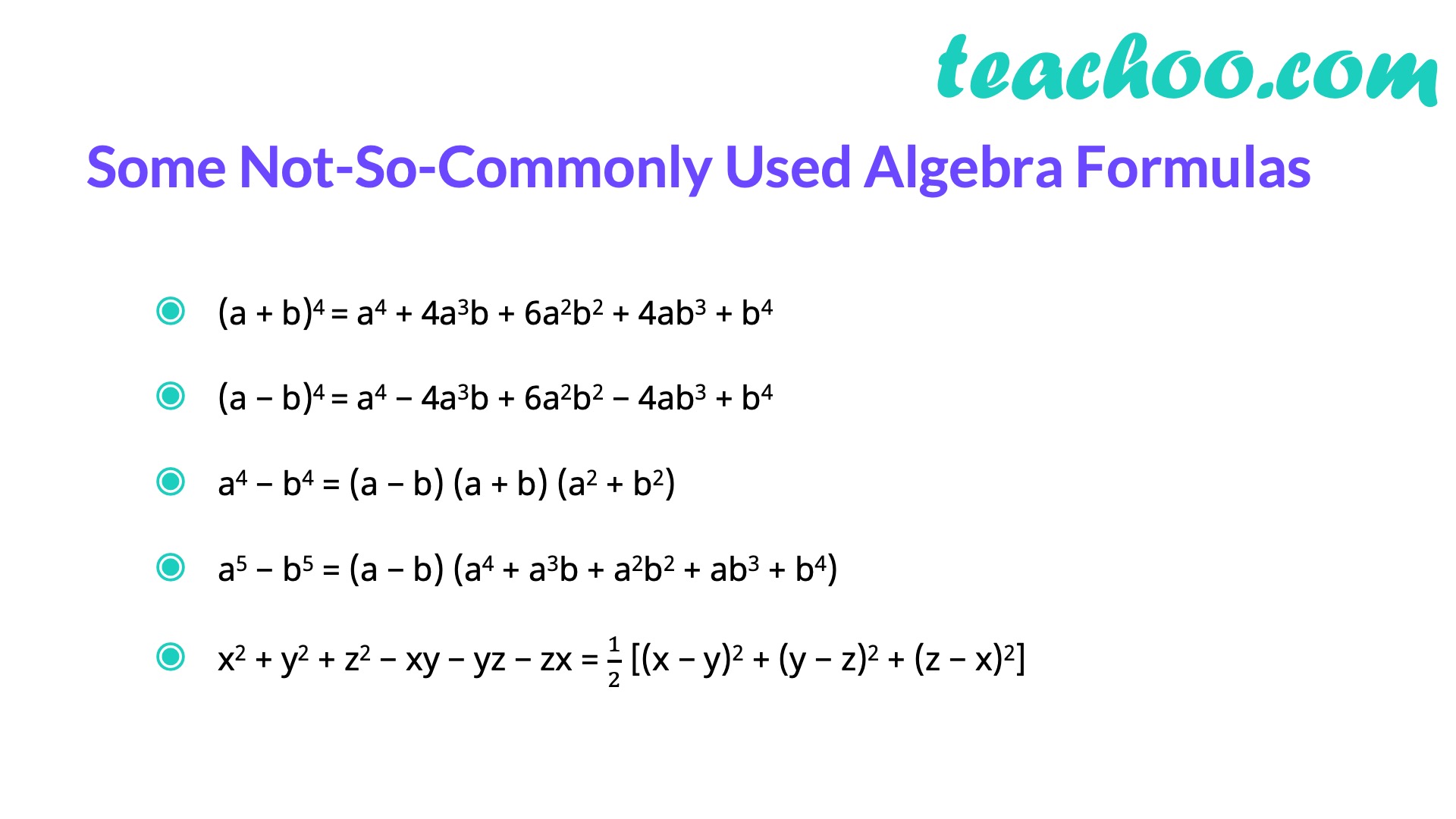
You will have 9(abc)>(abc)B(abc)/2 c(abc)/2 Therefore, semiperimeter is always greater than sides of the triangle and we cannot get any negative value under the root in Heron's formulaQuadratic Formula For an equation of the form \(ax^2bxc=0\), you can solve for x using the Quadratic Formula $$ x = \frac{b \pm \sqrt{b^24ac}}{2a} $$ Binomial Theorem \((ab)^1= a b\) \((ab)^2=a^22abb^2\) \((ab)^3=a^33a^2b3ab^2b^3\) \((ab)^4=a^44a^3b6a^2b^24ab^3b^4\) Difference of Squares \(a^2b^2=(ab)(ab)\) Rules of Zero


Search Q A3 2bb3 2bc3 Formula Tbm Isch



Formula Of A B C Whole Cube Math Polynomials Meritnation Com
The numerals a, b, and c are coefficients of the equation, and they represent known numbers For example, a cannot be 0, or the equation would be linear rather than quadratic A quadratic equation can be solved in multiple ways including Factoring, using the quadratic formula, completing the square, or graphingGet the list of basic algebra formulas in Maths at BYJU'S Stay tuned with BYJU'S to get all the important formulas in various chapters like trigonometry, probability and so onA and b are the other two sides ;


What Is The Perfect Formula For A B C Cube Quora


A 3 B 3 C 3 3abc Formula A B 2 A 2 B 2 2ab Proof Derivation Of Algebraic Identities Youtube Youtube
If a,b, and c are all real positive numbers then it will be correct But anyway to prove this without knowing anything factor out the 3 in the portion on the right You will have 27(abc)>3(abc), then divide both sides by 3;Example Solve 8a 3 27b 3 125c 3 – 90abc Solution This proceeds as Given polynomial (8a 3 27b 3 125c 3 – 90abc) can be written as (2a) 3 (3b) 3 (5c) 3 – 3(2a)(3b)(5c) And this represents identity a 3 b 3 c 3 3abc = (a b c)(a 2 b 2 c 2 ab bc ca) Where a = 2a, b = 3b and c = 5c Now apply values of a, b and c on the LHS of identity ie a 3 b 3 c 3History The formula is credited to Heron (or Hero) of Alexandria, and a proof can be found in his book, Metrica, written c CE 60 It has been suggested that Archimedes knew the formula over two centuries earlier, and since Metrica is a collection of the mathematical knowledge available in the ancient world, it is possible that the formula predates the reference given in that work
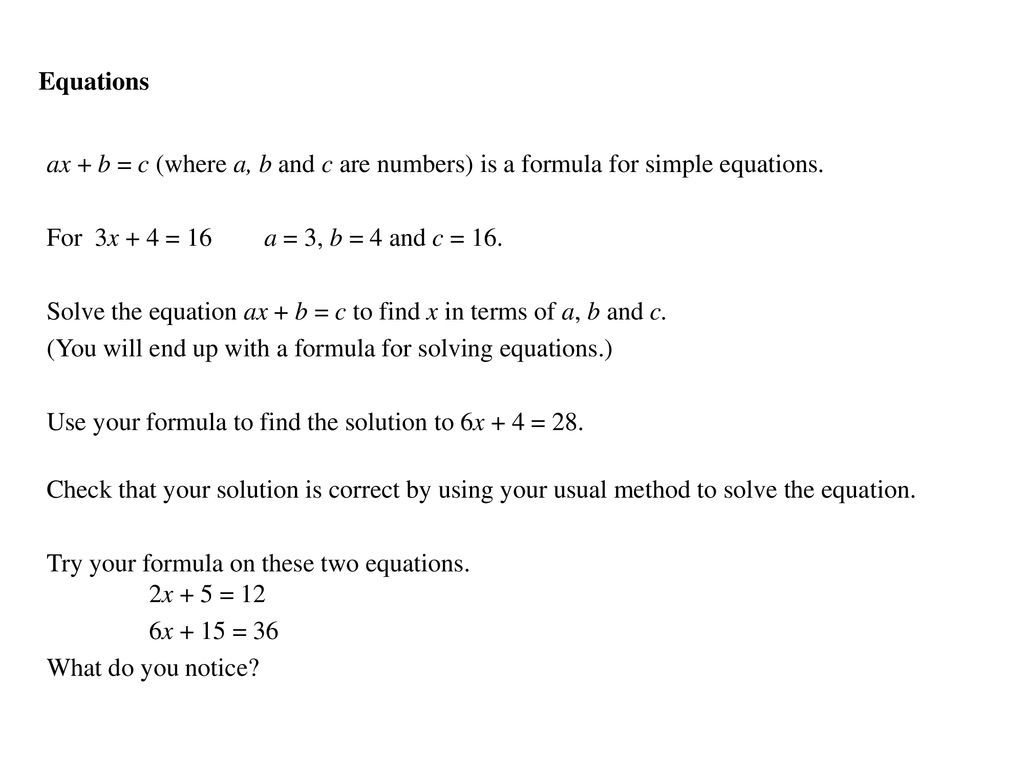


Solve The Equation Ax B C To Find X In Terms Of A B And C Ppt Download



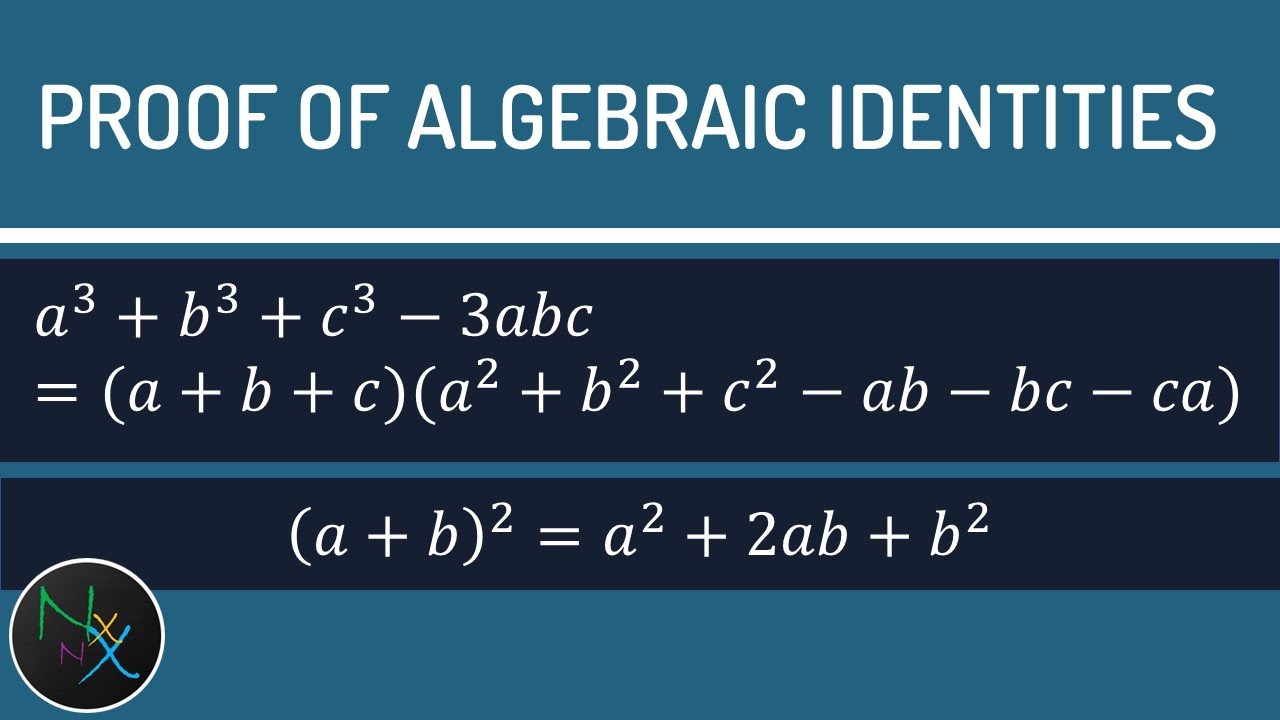
Prove A B C 3 A 3 B 3 C 3 3 A B B C C A Mathematics Topperlearning Com 9j3ovqff
A³ a²b a²c 2a²b 2ab² 2abc 2a²c 2abc 2ac² ab² b³ b²c 2abc 2b²c 2bc² ac² bc² c³ This is just multiplying out and bookkeeping It's a^3 b^3 c^3 plus 3 of eachQuite unfortunately not from the simple perspective requested, I have found that P = a 3 b 3 c 3 3abc = 2S, where S is the area of the triangle of vertices(a – b) 3 = a 3 – 3a 2 b 3ab 2 – b 3 (a b – c) 2 = a 2 b 2 c 2 2ab – 2bc – 2ca (a – b c) 2 = a 2 b 2 c 2 – 2ab – 2bc 2ca



Prove That A 3 B 3 C 3 3abc 1 2 A B C A B 2 B C 2 C A 2



Basic Formulae Square Formula 2 Variables
C 1C 3 C 3C 2 z z2 4 dz= Z C 1C 3 f 1(z) z 2i dz Z C 2 C 3 f 2(z) z 2i dz Since f 1 is analytic inside the simple closed curve C 1 C 3 and f 2 is analytic inside the simple closed curve C 2 C 3, Cauchy's formula applies to both integrals The total integral equals 2ˇi(f 1(2i) f 2( 2i)) = 2ˇi(1=2 1=2) = 2ˇi Remarks 1A 3 b 3 c 3 − 3abc = (a b c) (a 2 b 2 c 2 − ab − bc − ac) If (a b c) = 0, a 3 b 3 c 3 = 3abc Some not so Common FormulasSolving Quadratics by the Quadratic Formula – Pike Page 3 of 4 Example 3 Solve x2 25 = 8x 2Step 1 Simplify the problem to get the problem in the form ax bx c = 0 2x 25 = 8x → x2 – 8x 25 = 0 Step 2 Identify the values of a, b, and c, then plug them into the quadratic formula a = 1, b = –8, and c = 25 x= −b±√b2−4ac 2a
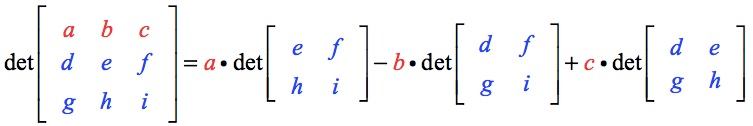


Determinant Of 3x3 Matrix Chilimath



Solved 410 Given Coplanar Vectors A B And C A 3 B 2 Chegg Com
The numerals a, b, and c are coefficients of the equation, and they represent known numbers For example, a cannot be 0, or the equation would be linear rather than quadratic A quadratic equation can be solved in multiple ways including Factoring, using the quadratic formula, completing the square, or graphingDefinition The longest side of the triangle is called the "hypotenuse", so the formal definition is5(a b c)2 = a2 b2 c2 2ab2bc 2ca 6(a b c)3 = a3 b3 c33a2b3a2c 3b2c 3b2a 3c2a 3c2a6abc 7a2 b2 = (a b)(a – b ) 8a3 – b3 = (a – b) (a 2 ab b2 ) 9a3 b3 = (a b) (a 2 ab b2 ) 10(a b)2 (a b) 2 = 4ab 11(a b)2 (a b) 2 = 2(a 2 b2 ) 12If a b c =0, then a3 b3 c3 = 3 abc INDICES AND SURDS 1 am a n = a m n 2
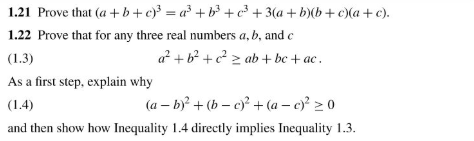


Solved 1 21 Prove That A B C 3 A3 C3 3 A B Chegg Com
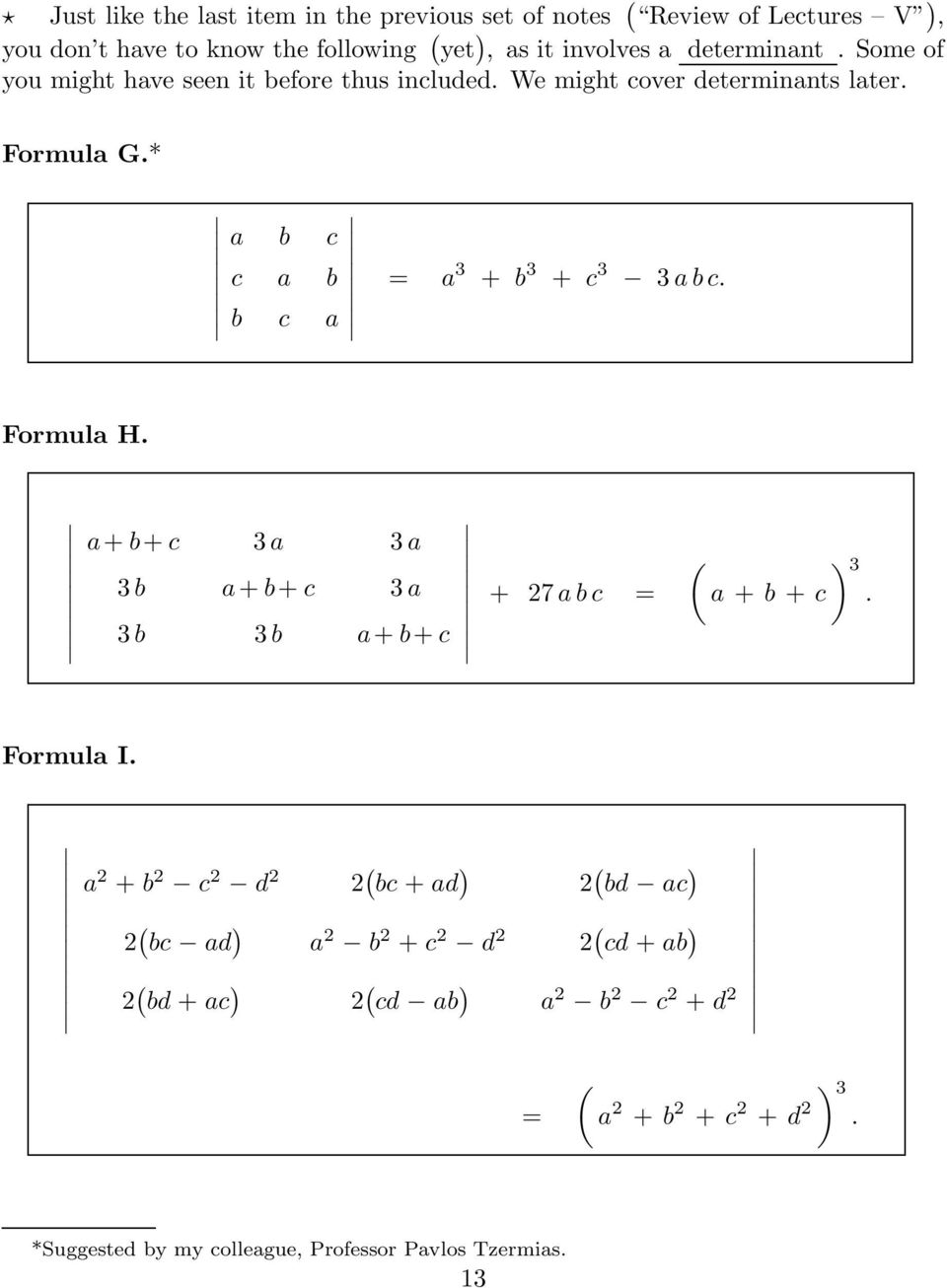


2 Two Cube 5 Five Cube 10 Ten Cube Pdf Free Download
Simplify the all Multiplication one by one \((abc)^3 = a \times (a^2b^2c^2 2ab 2bc 2ca)\\ b \times (a^2b^2c^2 2ab 2bc 2ca)\\ c \times (a^2b^2c^2 2ab 2bc 2ca) \) \(=> (abc)^3 = (a^3ab^2ac^2 2a^2b 2abc 2ca^2)\\ b \times (a^2b^2c^2 2ab 2bc 2ca)\\ c \times (a^2b^2c^2 2ab 2bc 2ca) \)Comments Have your say about what you just read!History The formula is credited to Heron (or Hero) of Alexandria, and a proof can be found in his book, Metrica, written c CE 60 It has been suggested that Archimedes knew the formula over two centuries earlier, and since Metrica is a collection of the mathematical knowledge available in the ancient world, it is possible that the formula predates the reference given in that work



Solved Question 2 A Given That In The Triangle Abc Ang Chegg Com



If A B C 5 And Ab Ca 10 Prove A 3 B 3 C 3 3abc 25 Cbse Class 9 Maths Learn Cbse Forum
Therefore, a 2 b 2 c 2 = 507 Thus, the formula of square of a trinomial will help us to expand 7th Grade Math Problems 8th Grade Math Practice From Square of a Trinomial to HOME PAGE New!Step 1 In this C program, User will enter the three sides of the triangle a, b, c Step 2 Calculating the Perimeter of the Triangle using the formula P = abc Step 3 Calculating the semi perimeter using the formula (abc)/2 Although we can write semi perimeter = (Perimeter/2) but we want to show the formula behind2 29 if a ib=0 wherei= p −1, then a= b=0 30 if a ib= x iy,wherei= p −1, then a= xand b= y 31 The roots of the quadratic equationax2bxc=0;a6= 0 are −b p b2 −4ac 2a The solution set of the equation is (−b p 2a −b− p 2a where = discriminant = b2 −4ac 32


What Is The Formula Of Math A 3 B 3 Math Quora
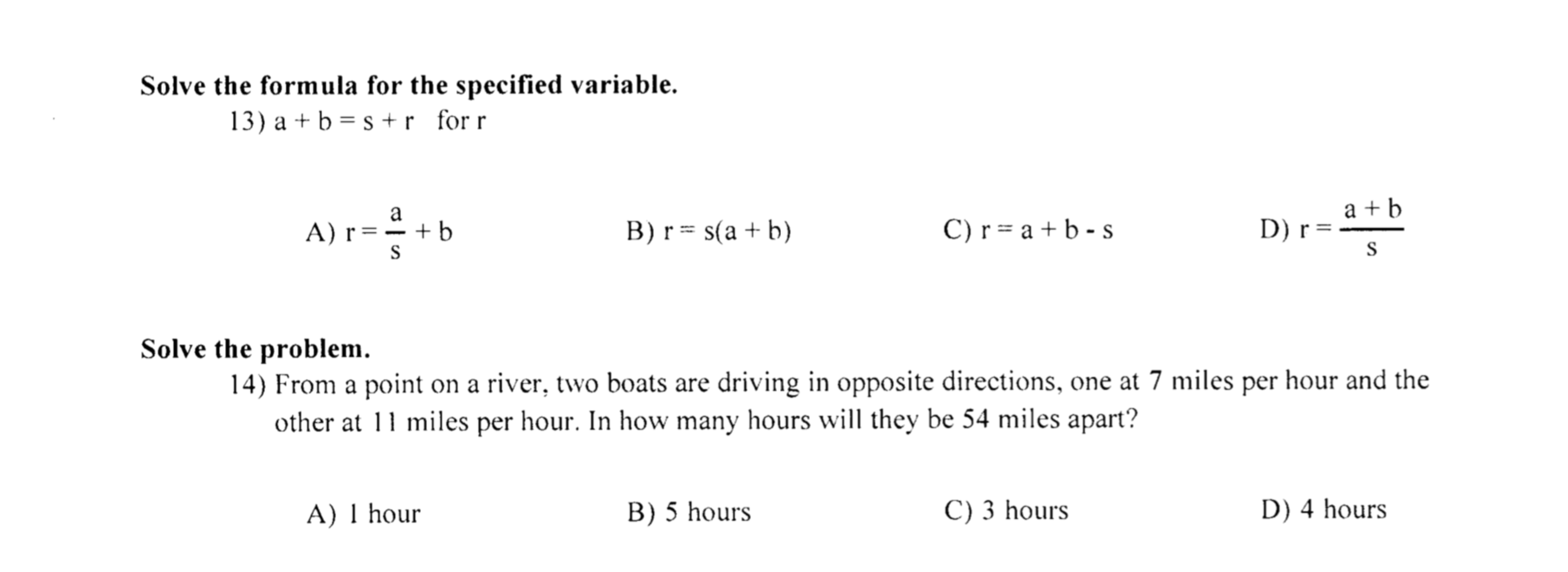


Solved Solve The Formula For The Specified Variable 13 Chegg Com
The Quadratic Formula will work with any quadratic equation, but only if the equation is in standard form, To use it, follow these steps · Put the equation in standard form first · Identify the coefficients, a, b, and c Be careful to include negative signs if the bx or c terms are subtracted · Substitute the values for the coefficients into the Quadratic FormulaA b c Add a on both the sides aa abc 2a abc a(abc)/2 (abc)/2 = semi perimeter You can also repeat the same for sides b and c Thus, you will get;If a,b, and c are all real positive numbers then it will be correct But anyway to prove this without knowing anything factor out the 3 in the portion on the right You will have 27(abc)>3(abc), then divide both sides by 3;


Blog Archives Eylemmath



Identities Math Methods Learning Mathematics Math Formula Chart
What is A3 formula a³ b³ = (a b)(a² – ab b²) you know that (a b)³ = a³ 3ab(a b) b³Calculator Use This online calculator is a quadratic equation solver that will solve a secondorder polynomial equation such as ax 2 bx c = 0 for x, where a ≠ 0, using the quadratic formula The calculator solution will show work using the quadratic formula to solve the entered equation for real and complex rootsYou will have 9(abc)>(abc)
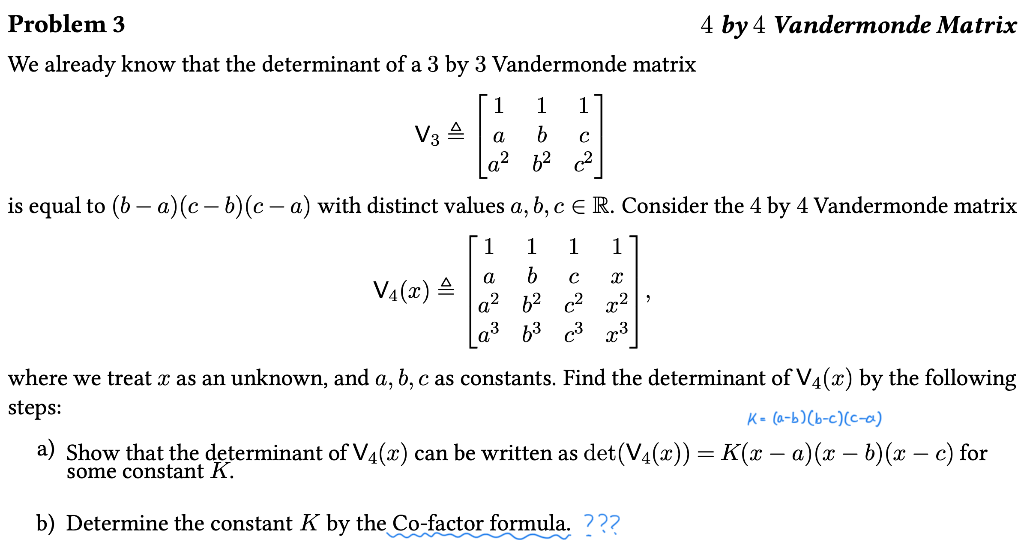


I Found K A B B C C A But What Is The Co Fa Chegg Com



Mathematical Formula For Competitive Exams Math Shortcut Tricks
Otherwise put, it is a product of sums or an AND of ORsAs a canonical normal form, it is useful in automated theorem proving and circuit theory All conjunctions of literals and all disjunctions of literals are inIt is called "Pythagoras' Theorem" and can be written in one short equation a 2 b 2 = c 2 Note c is the longest side of the triangle;Quadratic Formula For an equation of the form \(ax^2bxc=0\), you can solve for x using the Quadratic Formula $$ x = \frac{b \pm \sqrt{b^24ac}}{2a} $$ Binomial Theorem \((ab)^1= a b\) \((ab)^2=a^22abb^2\) \((ab)^3=a^33a^2b3ab^2b^3\) \((ab)^4=a^44a^3b6a^2b^24ab^3b^4\) Difference of Squares \(a^2b^2=(ab)(ab)\) Rules of Zero



Algebra Formulas A B 3 A B 2 A B C 3 A 3 B 3 Algebra Formulas Algebra Notes Algebra Help


A B C 3 Formula Expansion
Example has 2,a,b,c means that an entry must have at least two of the letters a, b and c The "no" rule which means that some items from the list must not occur together Example no 2,a,b,c means that an entry must not have two or more of the letters a, b and cQ = (x 2;y 2) you can obtain the following information 1The distance betweenQuadratics and Solving for x Quadratic Formula To solve ax2 bx c= 0, a6= 0, use x= 2b p b 4ac 2a The Discriminant The discriminant is the part of the quadratic equation under the radical, b2 4ac We use



A B C 3 Formula Proof



Multinomial Expansion
Recall the formula `(abc)^2 = a^2 b^2 c^2 2(ab bc ca)` Given that `a^2 b^2 c^2 = 250 , ab bc ca = 3 ` Then we have `(abc)^2 = a^2 b^2 c^2 2 (ab bcca)` `(abc)^2 = 250 2(3)` `(abc)^2 = 256` `(abc) =± 16`3 Quadratic Formula Finally, the quadratic formula if a, b and c are real numbers, then the quadratic polynomial equation ax2 bx c = 0 (31) has (either one or two) solutions x = b p b2 4ac 2a (32) 4 Points and Lines Given two points in the plane, P = (x 1;y 1);The right triangles constructed with the sides 'a', 'b' and 'c' are called Pythagorean triangles In this set of 3 integers, 'a' and 'b' represents the base and perpendicular whereas 'c' represents the measure of the hypotenuse So, according to the Pythagorean triples formula, it is a set of 3 positive integers such that



The Unit Cell Of A Compound Made Up Of The Three Elements X Y And Z Are Given Below Oo A X2yz3 B Xy3z C Xyz D X3yz2



Rbse Solutions For Class 12 Maths Chapter 13 Vector Ex 13 5 Class 12 Maths Studying Math 12th Maths
A3 b3 c3 = (a b c) (a2 b2 c2 – ab – bc – ca) 3abc My Mug (of) formulas Just another WordPresscom weblog « Inradius of a triangleA Pythagorean triple is a set of 3 positive integers for sides a and b and hypotenuse c that satisfy the Pythagorean Theorem formula a 2 b 2 = c 2 The smallest known Pythagorean triple is 3, 4, and 5



Perimeter Of A Triangle Definition Formula And Examples



Answered Ax B 3 Suppose F X Cx D A Find Bartleby
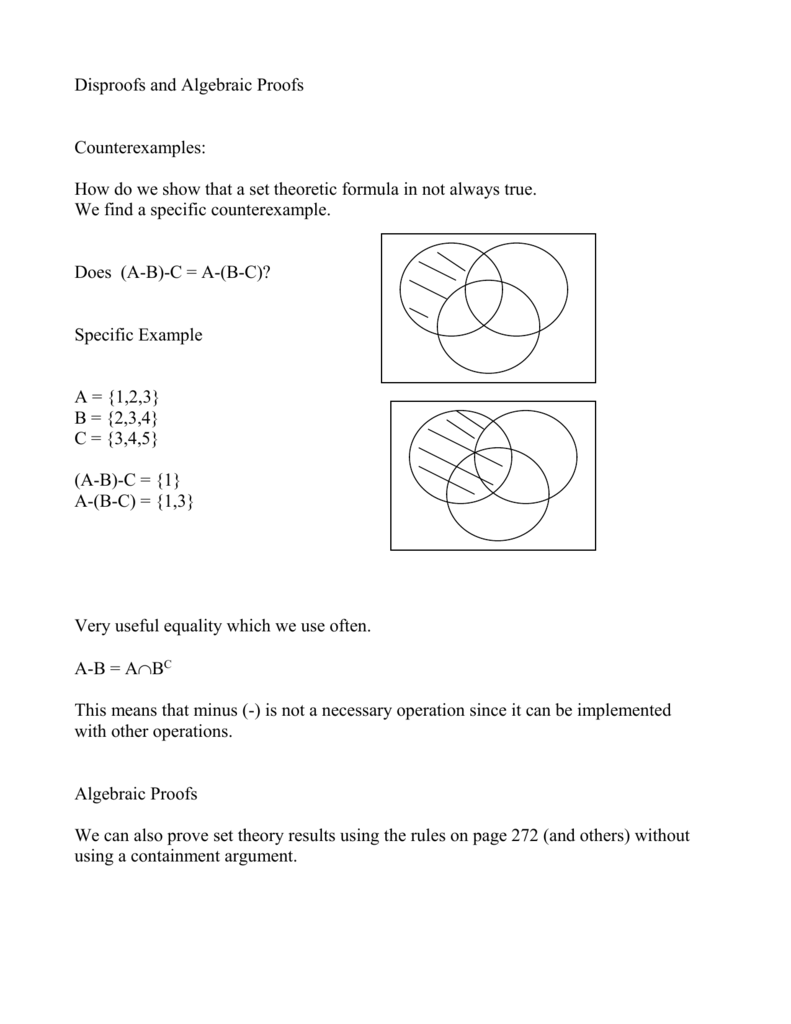


Discrete Mathematics Introduction


Evaluate The Formula Vectors A X A X C A C B A B C If Sarthaks Econnect Largest Online Education Community



Formula Centroid Of A Generalized Fuzzy Triangular Mathematics Stack Exchange
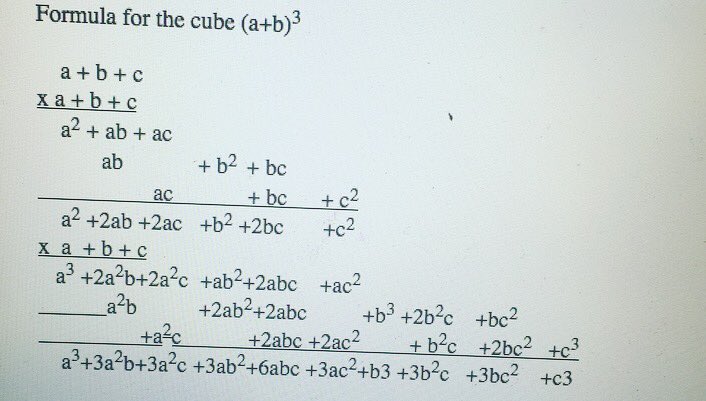


Eve Mendolia The Trinomial Cube Is A B C 3 Montessori Apsisawesome Apsdrew Apsvirginia Apsmath Suptpkm


Search Q A 5e2 2bb 5e2 Tbm Isch



Answered Let Bo B Bz Be The Sequence Bartleby
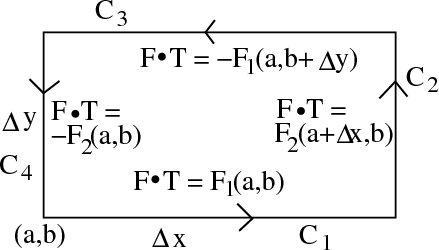


Calculating The Formula For Circulation Per Unit Area Math Insight


Prezentaciya Na Temu Chemistry 122 Introductory Organic Chemistry Fall Quarter 15 Dr Thomas H Schultz Skachat Besplatno I Bez Registracii
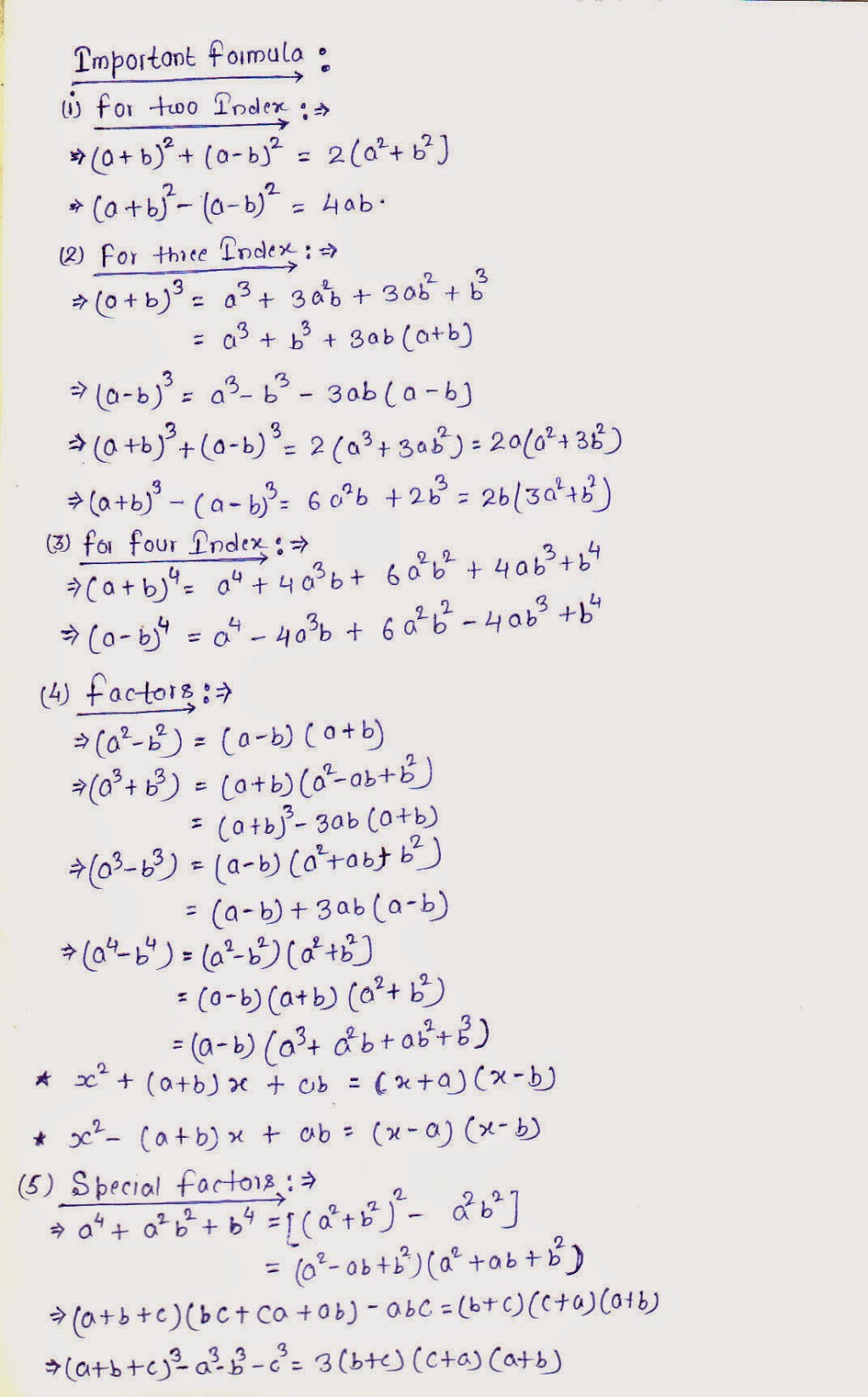


Important Tricks And Concept On Algebra



A 3 3 B 2 C 2 25 B 3 3 A 2 C 2 25 C 3 3 A 2 B 2 25 Find A B C Where A B C Are All Real Numbers Plz Solve This Hurry Sir Mathematics Topperlearning Com 4ko43xnn
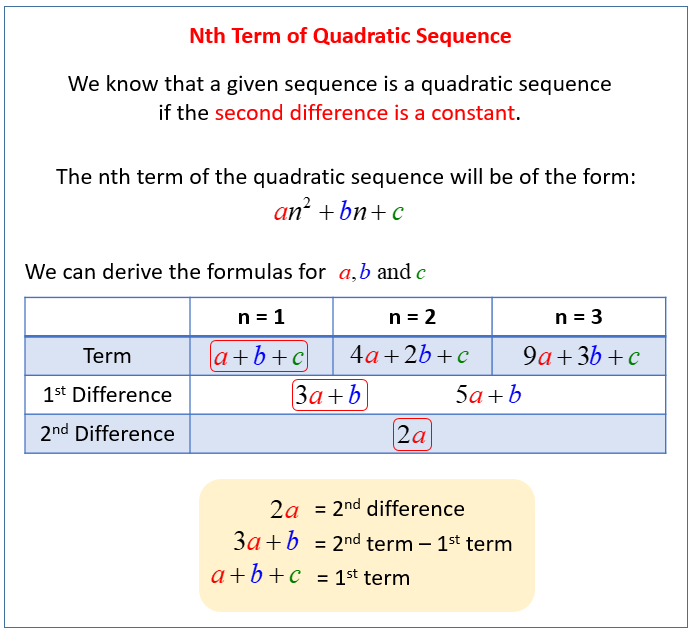


Quadratic And Cubic Sequences Solutions Examples Videos Worksheets Games Activities



What Is The Formula Of A B C Cube Math Algebraic Expressions And Identities Meritnation Com
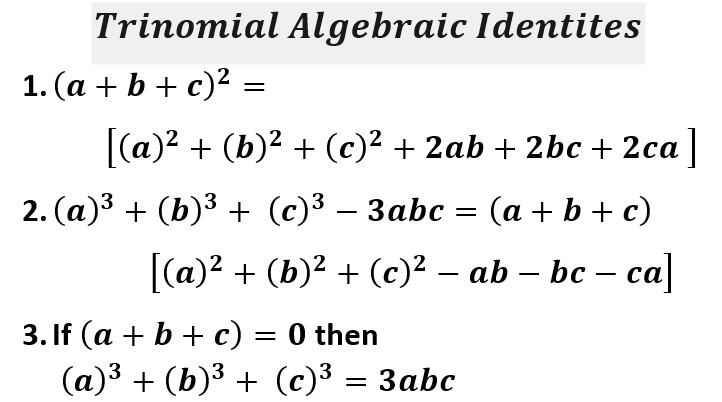


Mathematics Mathematics Formula Algebra Identities
.png)


All Mathematical Formula Chart The Future



How To Prove The Identiy A3 C3 3abc Math Polynomials Meritnation Com
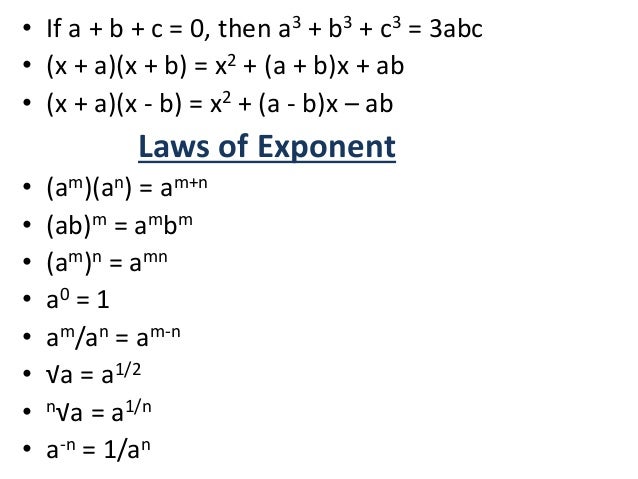


Mathematics Introduction And Formulas
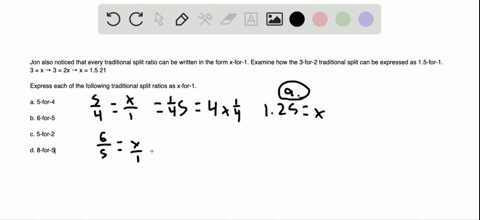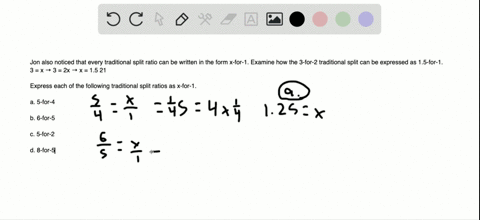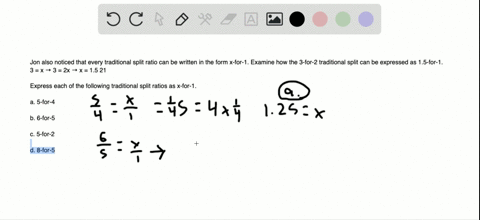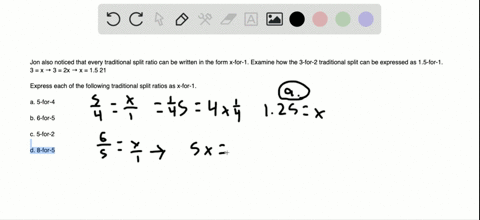


Solved Use The Following Spreadsheet To Answer Ex


Quadratic Formula Teacher Guide


With The Help Of The Flow Chart Given Below Solve The Equation X 2 2 3 X 3 0 Using The Formula Sarthaks Econnect Largest Online Education Community



Algebra Formula Definition Formulas And Examples
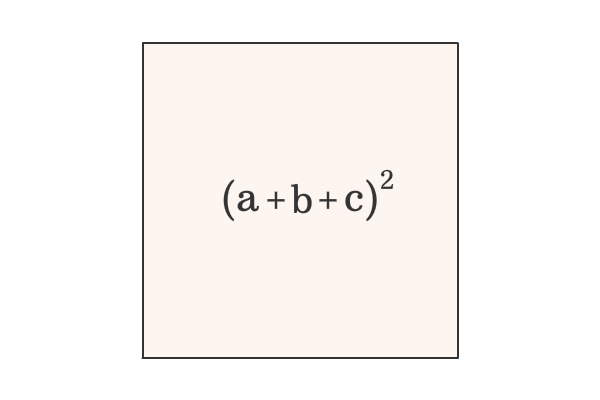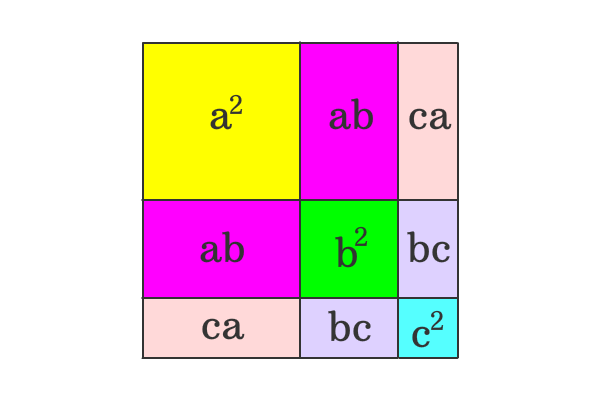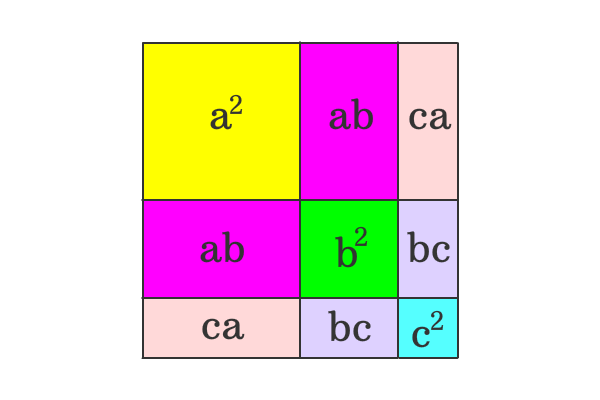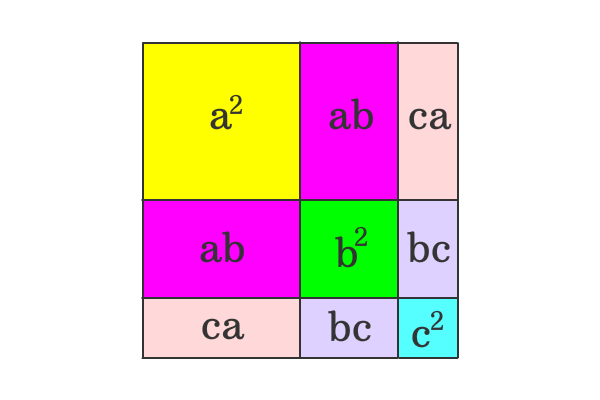


Proof Of A B C Formula In Geometric Method



If A B C 0 Then A B C 3 27abc How Mathematics Topperlearning Com 3wlz7ftt


Math Scene Equations Iii Lesson 2 Quadratic Equations



Prove That A B C 3 A 3 B 3 C 3 3 A B B C C A Youtube


Show That The Points A B C B C A C A B Are Collinear Coordinate Geometry Maths Class 10



Ex 12 3 4 Using Section Formula Show That Points A 2 3 4 B



Important Mathematical Formulas


Q Tbn And9gcrwxskagpy6lndt5stu6oxp1 K0tw6brabfeaf Ncwojlvhrcb Usqp Cau
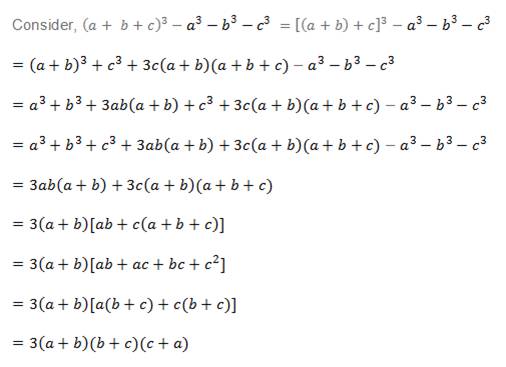


Prove That A B C 3 A3 C3 3 A B B C C A Polynomials Maths Class 9
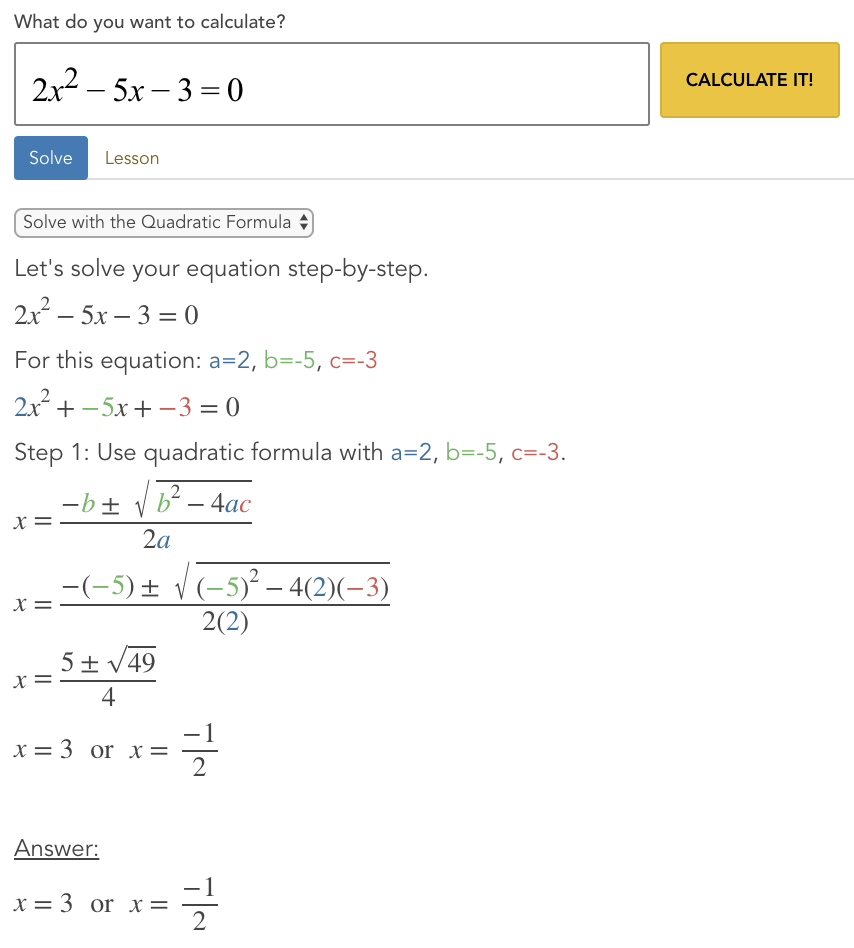


Quadratic Formula The Mathpapa Guide



Conventional Unit Cell Of E Carbide With A Fe 6 C 2 B Fe 12 C 5 Download Scientific Diagram



Algebra Problems Part 3 Questions Based On A B C 3abc A B C A B C Ab Ca Formula Youtube


What Is The Formula Of A B C A Whole Cube Quora



A 3 B 3 C 3 Formula Brainly In



Formulas For Generating Pythagorean Triples Wikipedia


If A B C 0 But Abc Don T Equal 0 Then A 2 B 2 Ca C 2 Ab Brainly Com


Http Www Mast Queensu Ca Mikeroth Oldteaching Linalg2 Hmwk Hmwk2 Pdf



If A 2 B 2 C 2 Ab Ca 0 A B C In R Then Find The Value O



What Are Various Forms To Write A B C A Whole Cube Quora


3 1 Functions A Relation Is A Set Of Ordered Pairs X Y Example The



What Is The Expansion Of A B C 3 Quora



Factorise A 3 B C 3 B 3 C A 3 C 3 A B 3
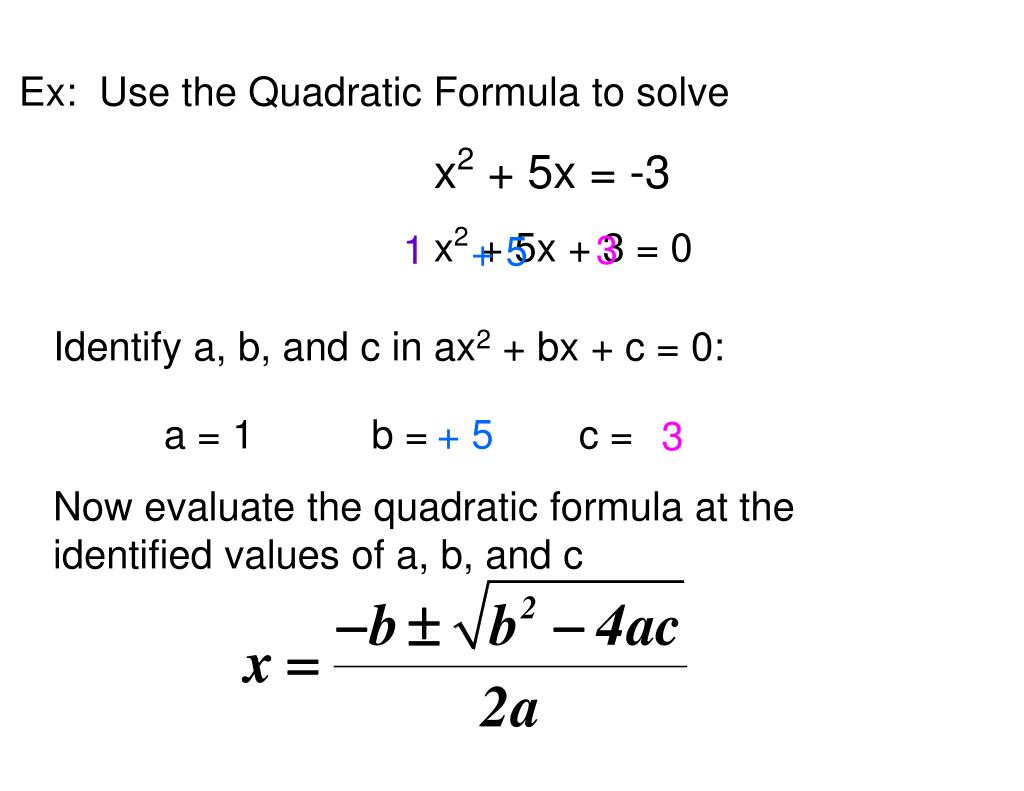


Ppt Quadratic Equations Powerpoint Presentation Free Download Id


New Page 1



How A 3 B 3 C 3 3abc Mathematics Topperlearning Com



Formula Of A3 C3 Brainly In
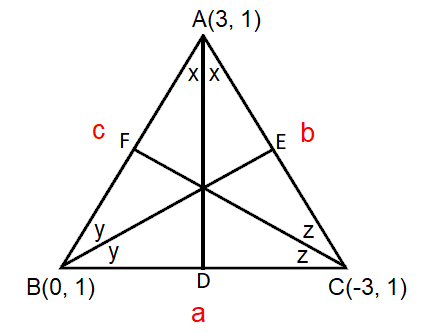


Incenter Of A Triangle


Solution Use The Binomial Theorem To Expand The Binomial And Express The Result In Simplified Form X 4 3



Optimized Structures Of Pristine C 3 N Along With Their Spin Density Of Download Scientific Diagram



In The Study Of Chlorination Of Propane Four Product A B C D



Yp Tutorials Important Formulas In Algebra Are You Aware Facebook


Q Tbn And9gcrwxskagpy6lndt5stu6oxp1 K0tw6brabfeaf Ncwojlvhrcb Usqp Cau



Toppershala Algebra Formulas Facebook
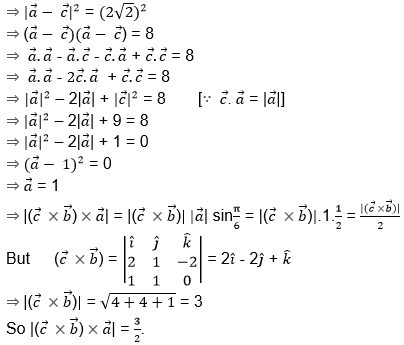


Vector Triple Product Definition Formula Proof Solved Problems



Algebra Formulas A B 3 A B 2 A B C 3 A 3 B 3 Teachoo


The Pythagorean Theorem



Question Prove That A B C 3 Ndash A3 C3 3 A B B C C A Mathematics Topperlearning Com We5wwhmm


Using Distance Formula Prove That The Following Points Are Collinear I A 4 3 1 B 5 7 6 And C 3 1 8 Sarthaks Econnect Largest Online Education Community



If The Roots Of The Equation A 2 X 2 2 B 2 Ca X C 2 Ab 0


Mathematics Article Village Of Valparaiso Home School Inc


Ssc Quantitative Aptitude Tricks Algebraic Equations



64 Useful Math Stations Teaching Info In Studying Math Basic Math Math Methods



Proof Of Formula Of Whole Cube Of A B C A B C 3 Youtube



Prove That Tex A B C 3 A 3 B 3 C 3 3 A B B C C A Tex Brainly In



I U 4 A B C A B Y 90 37 A Cubic Crystal Is Made



Proof Of Formula Of Whole Cube Of A B C A B C 3 Youtube



A B C 3 Expansion



3 C B



If A B C 0 Then A 3 B 3 C 3 0 How Quora
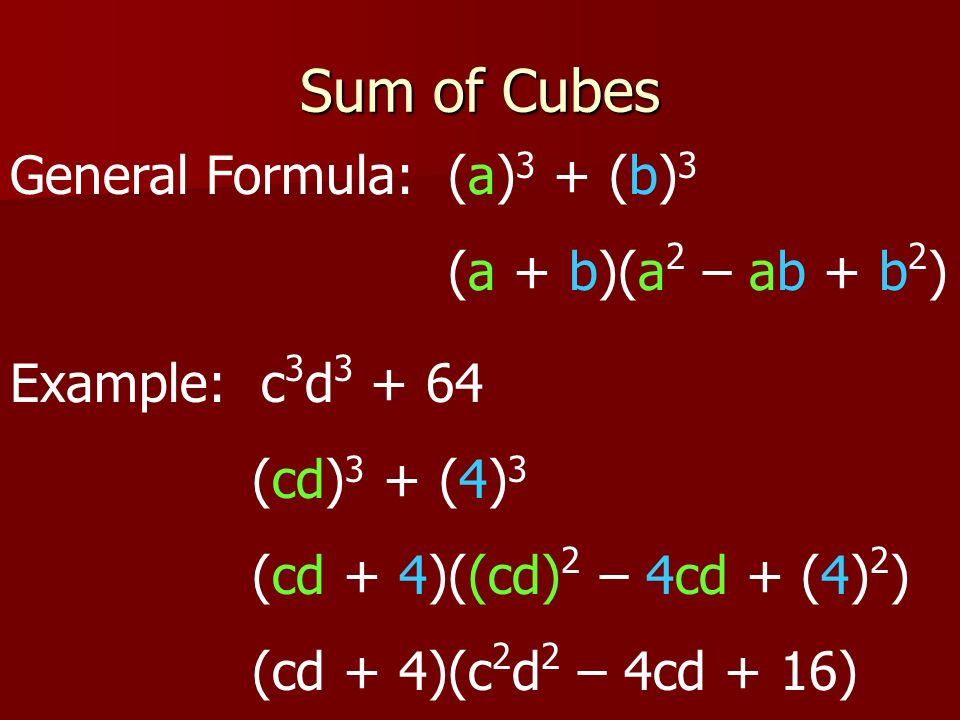


Tuesday July 1 Special Factoring Difference Of Squares Example M 2 64 M 2 8 2 M 8 M 8 Ppt Download



コメント
コメントを投稿